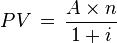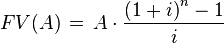Annuity Income Calculations
Time price of cash issues involve cyber web value of money flows at completely different points in time.
In a typical case, the variables may be: a balance (the real or par value of a debt or a money quality in terms of financial units), a periodic rate of interest, the amount of periods, and a series of money flows. (In the case of a debt, money flows area unit payments against principal and interest; within the case of a money quality, these area unit contributions to or withdrawals from the balance.) additional usually, the money flows might not be periodic however is also specific on an individual basis. Any of the variables is also the variable quantity (the sought-for answer) during a given downside. for instance, one could understand that: the interest is zero.5% per amount (per month, say); the amount of periods is sixty (months); the initial balance (of the debt, during this case) is twenty five,000 units; and therefore the final balance is zero units. The unknown variable is also the monthly payment that the recipient should pay.
For example, £100 endowed for one year, earning five-hitter interest, are value £105 once one year; so, £100 paid currently and £105 paid specifically one year later each have an equivalent price to a recipient UN agency expects five-hitter interest forward that inflation would be zero %. That is, £100 endowed for one year at five-hitter interest includes a future price of £105 underneath the belief that inflation would be zero %.
This principle permits for the valuation of a possible stream of financial gain within the future, in such the way that annual incomes area unit discounted and so extra along, so providing a lump-sum "present price" of the complete financial gain stream; all of the quality calculations for duration of cash derive from the foremost basic pure mathematics expression for this value of a future total, "discounted" to this by Associate in Nursing quantity adequate the duration of cash. for instance, the longer term price total FV to be received in one year is discounted at the speed of interest r to grant this price total PV:
PV = \frac
Some customary calculations supported the duration of cash are:
Present value: this value of a future total of cash or stream of money flows, given a specific rate of come. Future money flows area unit "discounted" at the discount rate; the upper the discount rate, the lower this price of the longer term money flows. decisive the suitable discount rate is that the key to valuing future money flows properly, whether or not they be earnings or obligations.
Present price of Associate in Nursing regular payment: Associate in Nursing annuity could be a series of equal payments or receipts that occur at equally spaced intervals. Leases and rental payments area unit examples. The payments or receipts occur at the top of every amount for a normal regular payment whereas they occur at the start of every amount for Associate in Nursing regular payment due.
Present price of a permanence is Associate in Nursing infinite and constant stream of identical money flows.
Future value: the worth of Associate in Nursing quality or money at a specific date within the future, supported the worth of that quality within the gift.
Future price of Associate in Nursing regular payment (FVA): the longer term price of a stream of payments (annuity), forward the payments area unit endowed at a given rate of interest.
There area unit many basic equations that represent the equalities listed higher than. The solutions is also found victimisation (in most cases) the formulas, a money calculator or a computer programme. The formulas area unit programmed into most money calculators and a number of other computer programme functions (such as PV, FV, RATE, NPER, and PMT).
For any of the equations below, the formula may additionally be rearranged to work out one in every of the opposite unknowns. within the case of the quality regular payment formula, however, there's no closed-form pure mathematics resolution for the rate (although money calculators and computer programme programs will without delay verify solutions through fast trial and error algorithms).
These equations area unit oftentimes combined for explicit uses. for instance, bonds may be without delay priced victimisation these equations. A typical bond certificate consists of 2 styles of payments: a stream of coupon payments kind of like Associate in Nursing regular payment, and a lump-sum come of capital at the top of the bond's maturity - that's, a future payment. the 2 formulas may be combined to work out this price of the bond.
An important note is that the rate i is that the rate for the relevant amount. For Associate in Nursing regular payment that creates one payment per annum, i'll be the annual rate. For Associate in Nursing financial gain or payment stream with a special payment schedule, the rate should be regenerate into the relevant periodic rate. for instance, a monthly rate for a mortgage with monthly payments needs that the rate be divided by twelve (see the instance below). See interest for details on changing between completely different periodic interest rates.
The rate of come within the calculations may be either the variable resolved for, or a predefined variable that measures a reduction rate, interest, inflation, rate of come, price of equity, price of debt or any range of alternative analogous ideas. the selection of the suitable rate is essential to the exercise, and therefore the use of Associate in Nursing incorrect discount rate can build the results unimportant.
For calculations involving annuities, you need to decide whether or not the payments area unit created at the top of every amount (known as a normal annuity), or at the start of every amount (known as Associate in Nursing regular payment due). If you're employing a money calculator or a computer programme, you'll be able to sometimes set it for either calculation. the subsequent formulas area unit for a normal regular payment. If you would like {the Associate in Nursingswer|the solution} for this price of an regular payment due merely multiply the PV of a normal regular payment by .
Formulas
The following formula use these common variables:
- PV is the value at time=0 (present value)
- FV is the value at time=n (future value)
- A is the value of the individual payments in each compounding period
- n is the number of periods (not necessarily an integer)
- i is the interest rate at which the amount compounds each period
- g is the growing rate of payments over each time period
Future value of a present sum
The future value (FV) formula is similar and uses the same variables.

Present value of a future sum
The present value formula is the core formula for the time value of money; each of the other formulae is derived from this formula. For example, the annuity formula is the sum of a series of present value calculations.
The present value (PV) formula has four variables, each of which can be solved for:
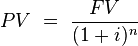
The cumulative present value of future cash flows can be calculated by summing the contributions of FVt, the value of cash flow at time t
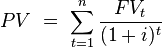
Note that this series can be summed for a given value of n, or when n is ∞. This is a very general formula, which leads to several important special cases given below.
Present value of an annuity for n payment periods
In this case the cash flow values remain the same throughout the n periods. The present value of an annuity (PVA) formula has four variables, each of which can be solved for:
![PV(A) \,=\,\frac{A}{i} \cdot \left[ {1-\frac{1}{\left(1+i\right)^n}} \right]](https://upload.wikimedia.org/math/c/b/6/cb63bc7899b0197bc65963ea0fe6e2f3.png)
To get the PV of an annuity due, multiply the above equation by (1 + i).
Present value of a growing annuity
In this case each cash flow grows by a factor of (1+g). Similar to the formula for an annuity, the present value of a growing annuity (PVGA) uses the same variables with the addition of g as the rate of growth of the annuity (A is the annuity payment in the first period). This is a calculation that is rarely provided for on financial calculators.
Where i ≠ g :
![PV\,=\,{A \over (i-g)}\left[ 1- \left({1+g \over 1+i}\right)^n \right]](https://upload.wikimedia.org/math/2/9/d/29def358b418103f96d8e8b50e016cfa.png)
Where i = g :
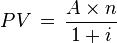
To get the PV of a growing annuity due, multiply the above equation by (1 + i).
Present value of a perpetuity
A perpetuity is payments of a set amount of money that occur on a routine basis and continues forever. When n → ∞, the PV of a perpetuity (a perpetual annuity) formula becomes simple division.

Present Value of Int Factor Annuity

Example:
- Investment P = $1000
- Interest i = 6.90% Compounded Qtrly (4 Times in Year)
- Tenure Years n = 5
Present value of a growing perpetuity
When the perpetual annuity payment grows at a fixed rate (g) the value is theoretically determined according to the following formula. In practice, there are few securities with precise characteristics, and the application of this valuation approach is subject to various qualifications and modifications. Most importantly, it is rare to find a growing perpetual annuity with fixed rates of growth and true perpetual cash flow generation. Despite these qualifications, the general approach may be used in valuations of real estate, equities, and other assets.
This is the well known Gordon Growth model used for stock valuation.
Future value of an annuity
The future value of an annuity (FVA) formula has four variables, each of which can be solved for:
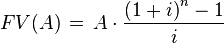
To get the FV of an annuity due, multiply the above equation by (1 + i).
Future value of a growing annuity
The future value of a growing annuity (FVA) formula has five variables, each of which can be solved for:
Where i ≠ g :

Where i = g :













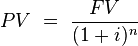
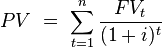
![PV(A) \,=\,\frac{A}{i} \cdot \left[ {1-\frac{1}{\left(1+i\right)^n}} \right]](https://upload.wikimedia.org/math/c/b/6/cb63bc7899b0197bc65963ea0fe6e2f3.png)
![PV\,=\,{A \over (i-g)}\left[ 1- \left({1+g \over 1+i}\right)^n \right]](https://upload.wikimedia.org/math/2/9/d/29def358b418103f96d8e8b50e016cfa.png)